Pythagorean theorem

In mathematics, the Pythagorean theorem or Pythagoras' theorem, is a relation in Euclidean geometry between the three sides of a right triangle.

The theorem is named after and commonly attributed to the 6th century BC Greek philosopher and mathematician Pythagoras, although the facts of the theorem were known by Indian (Baudhayana's and Katyayana's Sulbasutras), Greek, Chinese and Babylonian mathematicians well before he lived.

Two contemporary proofs can be considered the oldest record of the Pythagorean theorem: one to be found in Chou Pei Suan Ching (The Arithmetical Classic of the Gnomon and the Circular Paths of Heaven, ca. 500-200 B.C., see image below), the other in the Euclid's Elements.

Contents

1 The theorem 2 A visual proof 3 The converse 4 Generalizations 5 The Pythagorean theorem in non-Euclidean geometry 6 Heraldry 7 See also 8 External links

The theorem

The Pythagorean theorem states:

The sum of the areas of the squares on the legs of a right triangle is equal to the area of the square on the hypotenuse.

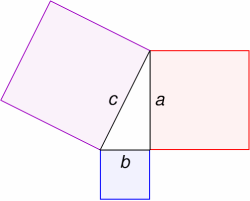
A right triangle is a triangle with one right angle; the legs are the two sides that make up the right angle, and the hypotenuse is the third side opposite the right angle. In the picture below, a and b are the legs of a right triangle, and c is the hypotenuse:

Pythagoras perceived the theorem in this geometric fashion, as a statement about areas of squares:

The sum of the areas of the blue and red squares is equal to the area of the purple square.

Similarly, the Indian Sulbasutra texts state that:

The rope which is stretched along the length of the diagonal of a rectangle produces an area which the vertical and horizontal sides make together.

Using algebra, one can reformulate the theorem into its modern expression by noting that the area of a square is the square (second power) of the length of its side:

Given a right triangle with legs of lengths a and b and hypotenuse of length c, then a² + b² = c².

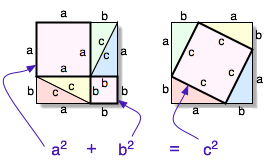
A visual proof

Perhaps this theorem has a greater variety of different known proofs than any other (the law of quadratic reciprocity may also be a contender for that distinction).

This illustration depicts one of them. The area of each large square is (a + b)². In both, the area of four identical triangles is removed. The remaining areas, a² + b² and c², are equal. Q.E.D.

NB: This proof is very simple, but it is not elementary, in the sense that it does not depend solely upon the most basic axioms and theorems of Euclidean geometry. In particular, while it is easy to give a formula for area of triangles and squares, it is not as easy to prove that the area of a square is the sum of areas of its pieces. In fact, proving the necessary properties is harder than proving the Pythagorean theorem itself. For this reason, axiomatic introductions to geometry usually employ another proof based on the similarity of triangles (see proof 6 in the external link).

There are many different proofs of the Pythagorean theorem; one was developed by James Garfield in 1876. Interestingly, Garfield would become President of the United States five years later. One of the proofs is based on Euler's formula in complex analysis. (See also the external links below for a sampling of the many different proofs of the Pythagorean theorem.)

Missing image
Chinese_pythagoras.jpg


Visual proof as in the Chou Pei Suan Ching 500-200 B.C.

The converse

The converse of the Pythagorean theorem is also true:

For any three positive numbers a, b, and c such that a² + b² = c², there exists a triangle with sides a, b and c, and every such triangle has a right angle between the sides of lengths a and b.

This converse also appears in Euclid's Elements. This can be proven using the law of cosines (see below under Generalizations).

It can also be proven by reductio ad absurdum. Suppose there exists a triangle for which a² + b² = c² but the angle between sides a and b is not a right angle. Then we can construct another triangle with a right angle between sides of lengths a and b. It follows that the hypotenuse of this triangle also has length c. Thus we have two triangles with the side lengths a, b and c but different angles between the a and b sides. But triangles with the same side lengths are congruent, and so we have a contradiction.

This section is a stub. You can help by adding to it (https://academickids.com:443/encyclopedia/index.php?title=Pythagorean_theorem&action=edit).

Generalizations

• The Pythagorean theorem was generalised by Euclid in his Elements:

If one erects similar figures (see Euclidean geometry) on the sides of a right triangle, then the sum of the areas of the two smaller ones equals the area of the larger one.

• The law of cosines (or cosine rule) is a version of the Pythagorean theorem that applies to all (Euclidean) triangles, not just right-angled ones. It states that

a² + b² + 2ab cos(θ) = c²,

where θ is the angle between sides a and b. When θ is 90 degrees, then cos(θ) = 0, so the formula reduces to the usual Pythagorean theorem.

• The Pythagorean theorem stated in Cartesian coordinates is the formula for the distance between points in the plane -- if (x₀, y₀) and (x₁, y₁) are points in the plane, then the distance between them is given by

<math> \sqrt{(x_1-x_0)^2 + (y_1-y_0)^2}. <math>

• More generally, given two vectors v and w in a complex inner product space, the Pythagorean theorem takes the following form:

<math>\|\mathbf{v}+\mathbf{w}\|^2 = \|\mathbf{v}\|^2 + \|\mathbf{w}\|^2 + 2\,\mbox{Re}\,\langle\mathbf{v},\mathbf{w}\rangle<math>

In particular, ||v + w||² = ||v||² + ||w||² if v and w are orthogonal, and these two statements are equivalent in any real inner product space.

• Using mathematical induction, the previous result can be extended to any finite number of pairwise orthogonal vectors. Let v₁, v₂,..., v_n be vectors in an inner product space such that <v_i, v_j> = 0 for 1 ≤ i < j ≤ n. Then

<math>\left\|\,\sum_{k=1}^{n}\mathbf{v}_k\,\right\|^2 = \sum_{k=1}^{n} \|\mathbf{v}_k\|^2<math>

The generalisation of this result to infinite-dimensional inner product spaces is known as Parseval's identity.

• The Pythagorean theorem also generalises to higher-dimensional simplexes. If a tetrahedron has a right angle corner (a corner of a cube), then the square of the area of the face opposite the right angle corner is the sum of the squares of the areas of the other three faces. This is called de Gua's theorem.

• Edsger Wybe Dijkstra discovered this generalisation:

sgn(α + β − γ) = sgn(a² + b² − c²)

where α is the angle opposite to side a, β is the angle opposite to side b and γ is the angle opposite to side c.

This formula holds in all triangles, not just the right triangles. If γ is a right angle (γ equals π/2 radians or 90°), then sgn(α + β − γ) = 0 since the sum of the angles of a triangle is π radians (or 180°). Thus, a² + b² − c² = 0.

In a triangle with three acute angles, α + β > γ holds. Therefore, a² + b² > c² holds.

In a triangle with an obtuse angle, α + β < γ holds. Therefore, a² + b² < c² holds.

The Pythagorean theorem in non-Euclidean geometry

The Pythagorean theorem is derived from the axioms of Euclidean geometry, and in fact, the Euclidean form of the Pythagorean theorem given above does not hold in non-Euclidean geometry. For example, in spherical geometry, all three sides of the right triangle bounding an octant of the unit sphere have length equal to π/2; this violates the Euclidean Pythagorean theorem because (π/2)² + (π/2)² ≠ (π/2)².

This means that in non-Euclidean geometry, the Pythagorean theorem must necessarily take a different form from the Euclidean theorem. There are two cases to consider -- spherical geometry and hyperbolic plane geometry; in each case, as in the Euclidean case, the result follows from the appropriate law of cosines:

• For any right triangle on a sphere of radius R, the Pythagorean theorem takes the form

<math> \cos \left(\frac{c}{R}\right)=\cos \left(\frac{a}{R}\right)\,\cos \left(\frac{b}{R}\right).<math>

By using the Maclaurin series for the cosine function, it can be shown that as the radius R approaches infinity, the spherical form of the Pythagorean theorem approaches the Euclidean form.

• For any triangle in the hyperbolic plane (with Gaussian curvature −1), the Pythagorean theorem takes the form

<math> \cosh c=\cosh a\,\cosh b<math>

where cosh is the hyperbolic cosine. By using the Maclaurin series for this function, it can be shown that as a hyperbolic triangle becomes very small (i.e., as a, b, and c all approach zero), the hyperbolic form of the Pythagorean theorem approaches the Euclidean form.

Heraldry

In heraldry the Pythagorean theorem appears as a charge in the arms of Seissenegger.

See also

• Baudhayana
• Katyayana
• Euclid
• Fermat's last theorem
• linear algebra
• orthogonality
• Pythagoras
• Pythagorean triple
• parallelogram law
• synthetic geometry

External links

• Several proofs of the Pythagorean theorem (http://www.cut-the-knot.org/pythagoras/index.html)
• Using the Pythagorean theorem and a Microsoft Windows calculator to calculate the sides of an octagon (http://buster2058.netfirms.com/octagon/octagon.htm)
• Dijkstra's generalization (http://www.cs.utexas.edu/users/EWD/ewd09xx/EWD975.PDF)
• The Pythagorean Theorem is Equivalent to the Parallel Postulate. (http://www.cut-the-knot.org/triangle/pythpar/PTimpliesPP.shtml)ar:نظرية فيثاغورث

bg:Питагорова теорема ca:Teorema de Pitàgores de:Satz des Pythagoras es:Teorema de Pitágoras fr:Théorème de Pythagore gl:Teorema de Pitágoras id:Teorema Pythagoras io:Teoremo di Pitagoro is:Pýþagórasarreglan it:Teorema di Pitagora he:משפט פיתגורס lt:Pitagoro teorema lv:Pitagora teorēma hu:Pitagorasz-tétel nl:Stelling van Pythagoras ja:ピタゴラスの定理 pl:Twierdzenie Pitagorasa pt:Teorema de Pitágoras ru:Теорема Пифагора scn:Tiurema di Pitagora sl:Pitagorov izrek sr:Питагорина теорема fi:Pythagoraan teoreema sv:Pythagoras sats th:ทฤษฎีบทพีทาโกรัส tr:Pisagor teoremi zh:勾股定理